Panorama Photography

Lihi Zelnik-Manor, Gabriele Peters, Pietro Perona

Publication:

• Lihi Zelnik-Manor, Gabriele Peters, and Pietro Perona,
  Squaring the Circle in Panoramas, 10th IEEE International Conference on Computer Vision (ICCV 2005), IEEE Computer Society, pp. 1292-1299, Beijing, China, October 15-21, 2005.

All publications are available from Publications.

Abstract

Pictures taken by a rotating camera cover the viewing sphere surrounding the center of rotation. Having a set of images registered and blended on the sphere what is left to be done, in order to obtain a flat panorama, is projecting the spherical image onto a picture plane. This step is unfortunately not obvious - the surface of the sphere may not be flattened onto a page without some form of distortion. The objective of this paper is discussing the difficulties and opportunities that are connected to the projection from viewing sphere to image plane. We first explore a number of alternatives to the commonly used linear perspective projection. These are `global' projections and do not depend on image content. We then show that multiple projections may coexist successfully in the same mosaic: these projections are chosen locally and depend on what is present in the pictures. We show that such multi-view projections can produce more compelling results than the global projections.

Summary

Images taken by a rotating camera can be registered and blended on the sphere using available software tools (e.g. Autostitch). To obtain a single flat image one has to project the sphere onto the plane. This projection is bound to introduce some perceptual distortions in the resulting panorama. The two most common projections used for panoramas are perspective and geographic. Many other projections have been suggested by cartographers but all introduce some perceptual distortions.

Perspective Projection

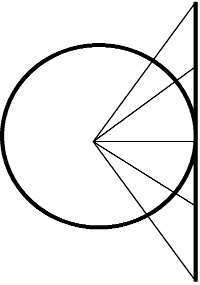
• - cannot handle wide fields of view as the projection plane size runs to infinity
• - distorts objects at large viewing angles

cubes under perspective projection at wide field of view

overlay of rectified face images taken by a rotating camera

Geographic Projection

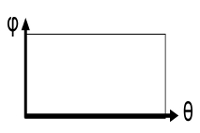
• - This is a cylindrical projection where the horizontal axis is linearly proportional to the horizontal angle and the vertical axis is linearly proportional to the vertical angle (same proportion for both axes).
• - It bends horizontal scene lines.

Mercator Projection

A conformal cylindrical projection. The horizontal axis is linearly proportional to the horizontal angle. The vertical axis is set to maintain conformality, i.e., local aspect proportions are maintained and small circles in the scene are projected onto circles in the panorama. This is different from the geographic projection which turns circles into ellipses.

Geographic projection: The shaft of the Pantheon ceiling is squished into an ellipse

Mercator projection: The shaft circularity is maintained

Comparison of the Three Projections

Perspective projection: stretches too much

Geographic projection: bends horizontal scene lines

Mercator projection: bends horizontal scene lines

Suggested Alternative: Multi-Plane Perspective Projection

Project the sphere onto multiple planes which are then unfolded into a single flat image. The projection onto each plane is perspective. The large discontinuities between the planes can be hidden where the world already has a discontinuity.

Preserving Foreground Objects

Foreground (nearby, viewed at large angle) objects look distorted under perspective projection. To correct for that we allow multiple view points to reside in the same panorama. This is done by using a different projection for different objects in the scene.